|
ISSN: 2951-6625
Nº 145 (3 de octubre de 2024)
|

|
1) Activities from October 3 to 11, 2024
Seminario de Análisis Matemático y Matemática Aplicada
Title: Oscillatory behavior in coagulation-fragmentation models
Speaker: Juan José López Velázquez (Institute for Applied Mathematics, University of Bonn)
Day: October 3, 2024
Place: Seminario Alberto Dou (Room 209)
Hour: 13:00
Organized by: Departamento de Análisis Matemático y Matemática Aplicada and Instituto de Matemática Interdisciplinar (IMI)
Seminario de Doctorandos
Título: Fenomenología (matemática) de las ecuaciones de Euler (y otras EDPs)
Doctorando: Andrés Laín Sanclemente (ICMAT)
Día: 3 de octubre, 2024
Lugar: Seminario Alberto Dou (209)
Hora: 17:00
Organizado por: Facultad de Ciencias Matemáticas UCM y Red de Doctorandos UCM, con la colaboración del Instituto de Matemática Interdisciplinar (IMI)
I MaPhyAG (Mathematical Physics and Algebraic Geometry) Workshop: Integrability, Geometry and QFT
Speakers: Mirjam Cvetic (UPenn), Tudor Dimofte (U Edinburgh), Jacques Distler (UT Austin), Ron Donagi (UPenn), Olivia Dumitrescu (U North Carolina Chapel Hill), Sergei Gukov (Caltech), Sungkyung Kang (U Oxford), Craig Lawrie (DESY), Marta Mazzocco (UP Cataluña), Motohico Mulase (UC Davis), Ana Péon-Nieto (U Santiago de Compostela), Laura Schaposnik (U Illinois Chicago), Eric Sharpe (Virginia Tech) and Szilárd Szabó (Alfréd Rényi Institute of Mathematics).
Date: October 7-11, 2024.
Hour: 9:00h
Place: Assembly Hall M2, Facultad CC. Físicas, Universidad Complutense de Madrid
Organized by: Enrique Arrondo (UCM-IMI), Ángel González-Prieto (UCM-ICMAT), Monica Jinwoo Kang (UPenn), Marina Logares (UCM-IMI), Piergiulio Tempesta (UCM-ICMAT)

Seminario de Análisis Matemático y Matemática Aplicada
Title: Strongly norm attaining Lipschitz maps
Speaker: Miguel Martín (Universidad de Granada)
Day: October 10, 2024
Place: Seminario Alberto Dou (Room 209)
Hour: 13:00
Organized by: Departamento de Análisis Matemático y Matemática Aplicada and Instituto de Matemática Interdisciplinar (IMI)
P. Terán-Viadero, A. Alonso-Ayuso, F. Javier Martín-Campo. Mathematical optimisation in the honeycomb cardboard industry: A model for the two-dimensional variable-sized cutting stock problem. European Journal of Operational Research, 319, (1), 303-315. 2024. DOI: 10.1016/j.ejor.2024.06.022.
A. García, M. Negreanu, F. Ureña, A. M. Vargas. Numerical solution of a hydrodynamic model with cavitation using finite difference method at arbitrary meshes. Computational Particle Mechanics. 205, 195-205. 2024. DOI: 10.1016/j.apnum.2024.07.007.
3) Other planned activities
Seminario de Álgebra, Geometría y Topología
Título: Criptografía con y contra las tecnologías cuánticas
Oradora: María Isabel González Vasco (Universidad Carlos III de Madrid)
Fecha: 15 de octubre, 2024
Lugar: Seminario 238
Hora: 13:00
Organizado por: Departamento de Álgebra, Geometría y Topología e Instituto de Matemática Interdisciplinar (IMI)
Seminario de Análisis Matemático y Matemática Aplicada
Título: Sistemas Integrables, controlabilidad y observabilidad
Orador: Raúl Felipe (CIMAT - Guanajuato, México)
Fecha: 24 de octubre, 2024
Lugar: Seminario Alberto Dou (Aula 209)
Hora: 13:00
Organizado por: Departamento de Análisis Matemático y Matemática Aplicada and Instituto de Matemática Interdisciplinar (IMI)
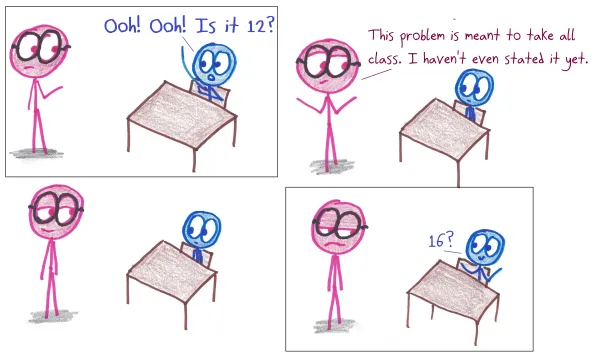
4) La viñeta matemática
Comic strip sent by Ben Orlin and used with permission.
5) Math Puzzle
Puzzle sent by Kjartan Poskitt.
The solution will be provided in the next issue of Boletin del IMI.
Solution to last issue's Math Puzzle, sent by Rik Tangerman and published on issue No. 144 of the Boletín del IMI:
The eye mask
The circle area is 2πAB/(A+B).
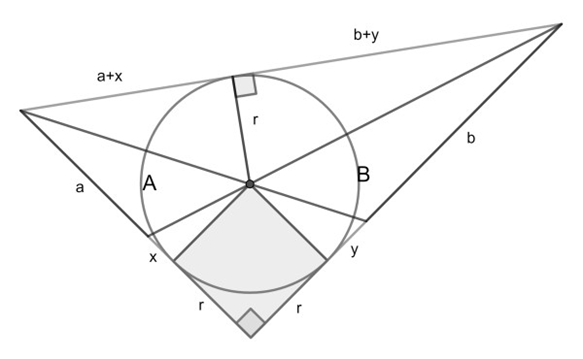
First note that the two triangles both have height r, which is also the circle radius. Name their respective bases a and b. The two small triangles have bases x and y.
From triangle similarity one can show that x/r=r/(b+y) and y/r=r/(a+x). Eliminating r from these two equations leads to x/y=a/b. So we get that b+y=(a+x)b/a=bz/a, where we temporarily named a+x=z. Filling this back in we get that r2=yz=xbz/a.
Now the total area is (r+z)(r+bz/a)/2. By adding up the area of the square and the four right triangles we get that this must be equal to r2+rz+rzb/a. After some algebra, also using the equation for r2, we get xb/a+r+rb/a-bz/a=0. Eliminating z, this leads to r=ab/(a+b).
Finally, using A=ar/2 and B=br/2, we reach the desired formula.
6) Math Art
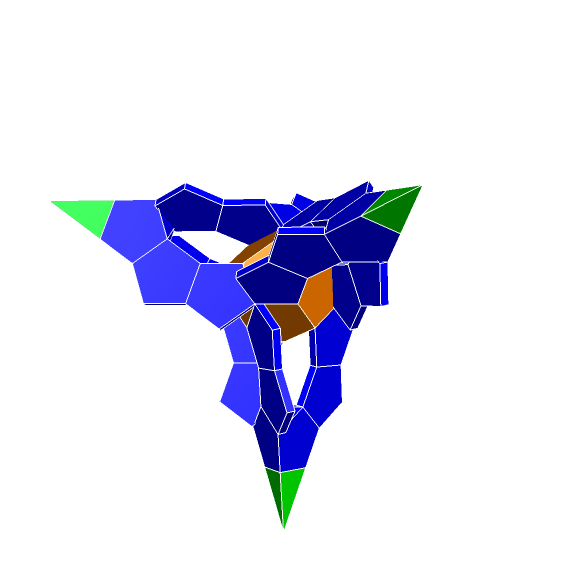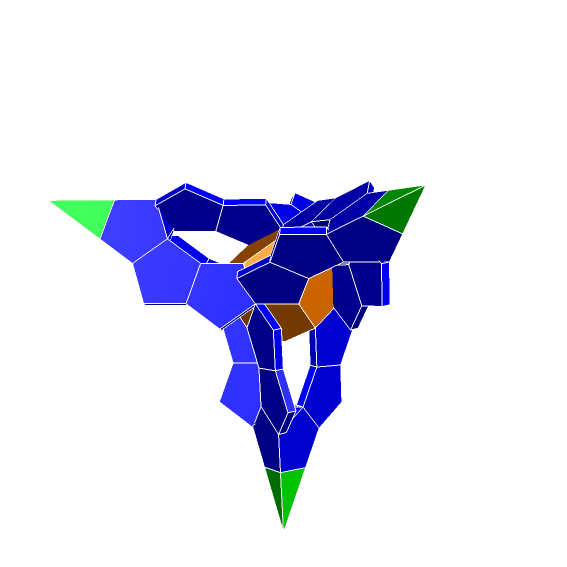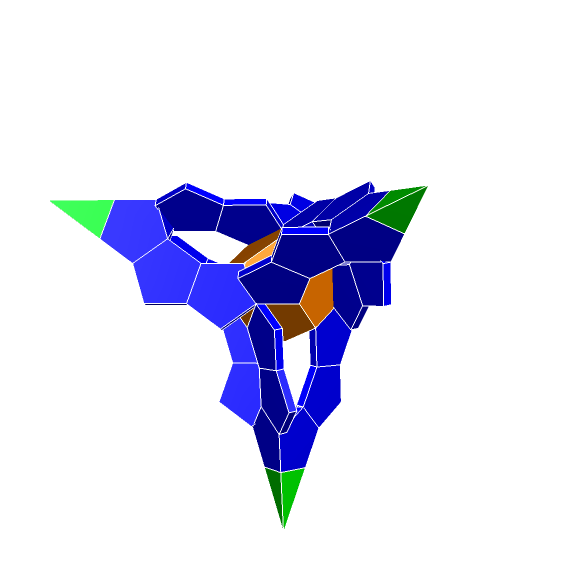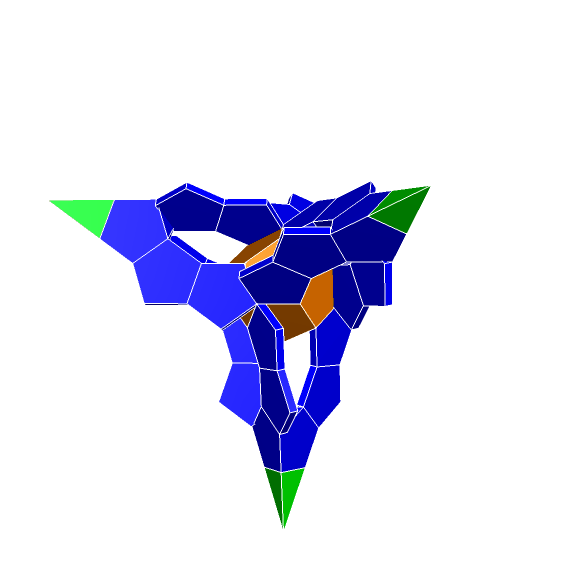
Escher Tetrahedral Polyknot